In a class of 40 students, each student offers at least one of physics and chemistry. If the number of students that offer physics is three times the number that offer both subjects, and the number that offer chemistry is twice the number that offer physics, find the number of students that offer physics only.
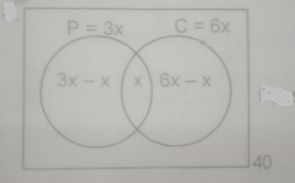
Let the number of students that offer both subject be x
hence, the number of students offering physics = 3x
The number of students offering chemistry = 6x
The number of students offering physics only = 3x - x = 2x
The number of students offering chemistry only = 6x - x = 5x
So, 2x + 5x + x = 40
8x = 40
x = \(\frac{40}{8}\) = 5.
Therefore, the number of students offering physics only = 2x = 2 x 5 = 10.
Contributions ({{ comment_count }})
Please wait...
Modal title
Report
Block User
{{ feedback_modal_data.title }}