Two observers Abu and Badu, 46 m apart, observe a bird on a vertical pole from the same side of the bird. The angles of elevation of the bird from Abu's and Badu's eye are 40º and 48º respectively. If at the foot of the pole Abu and Badu are on same horizontal;
(a) illustrate the information in a diagram;
(b) calculate, correct to one decimal place, the height of the pole.
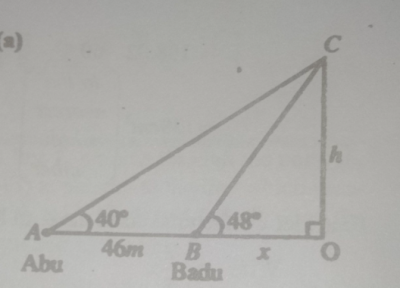
(a) The diagram above is the illustration of the given information.
(b) Let |OB| = x, and |OC| = h
From \(\triangle\)OBC
Tan 48º = \(\frac{h}{x}\)
h = x tan48 - - - - - - - - - - - - -(i)
From \(\triangle\)AOC
tan 40º = \(\frac{h}{46 + x}\)
h = tan 40(46 + x) = 46(tan40) + x(tan40) - - - - - - - - - - -(ii)
Equate -- - - -eqn (i) and (ii)
x tan 48 = 40(tan46) + x(tan40)
x tan 48 - x tan 40 = 40 tan 46
x(tan 48 - tan 40) = 40 tan 46
x(1.1106 - 0.8391) = 38.5986
x(0.2715) = 38.5986
x = \(\frac{38.5986}{0.2715}\) = 142.1680
Height of pole = from eqn (i) = 142.1680 x 1.1106 = 157.8918 ≈ 157.9 m ( to one decimal place)
Contributions ({{ comment_count }})
Please wait...
Modal title
Report
Block User
{{ feedback_modal_data.title }}